If a body is thrown up in the air such that it deviates from simple one dimensional motion, it is termed a projectile. The analysis of motion associated with a projectile is known as projectile motion The path followed by a projectile is called its trajectory, which is directly influenced by gravity.
The initial velocity v0 can be written as
Since there is no acceleration in the horizontal direction, the horizontal component of the velocity remains unchanged throughout the motion.
The vertical motion is the motion of a particle in free fall. Equations for free fall apply. For example,
 . Other useful equations for the vertical y-axis are
. Other useful equations for the vertical y-axis are  , and
, and  .
.Eliminating t between the following two equations,
 and
and  , we obtain the equation of the path (the trajectory) of the projectile:
, we obtain the equation of the path (the trajectory) of the projectile:












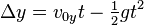





0 comments:
Post a Comment